40
How is it possible to sail from the UK to New Zealand along a very nearly straight line without intercepting any landmasses?
(hexbear.net)
It's a Bluesky link but he use adult tags as de facto "spoiler" tags. The problem is that content is hidden if you're not logged into Bluesky. Here's a Bluesky mirror site to show the entire thread...
https://subium.com/profile/c0nc0rdance.bsky.social/post/3kpkcq2ecws22
A huge hint...
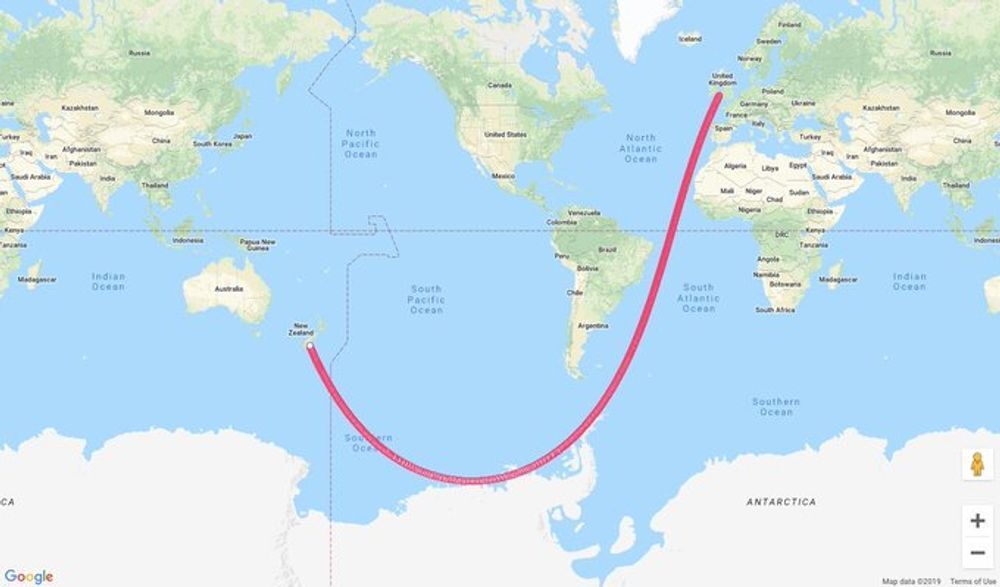
My point is that exactly. We live and move in 3D space, so the line has to be judged in 3 dimensions. You might as well say any curved line on a 2D map looks curved, but if you look at it in 1D it is perfectly straight
No we dont live in a 3d space. That's a mathematical model used to model reality so as to be able to ignore details deemed unecessary for whatever the model is for. It's a tool to approximate reality not reality itself.
And for the purposes of traversing our globe a 3rd dimension is unecessary so why include that in your model?
And even if, its blatantly obvious that the OOP is asking for a straight line in a 2d perspective, not on a map, but on the globe itself because any projection of a globe into a flat space will take the straightness out of a straight line.
How would you begin to describe points in the spaces we are discussing? I feel this is a fair question, because in an earlier reply you suggest to picking a point and walking there.
For the surface of a sphere, the most natural way many people would choose to do this would be using the tuples (x,y,z) in R^3^ and restricting this space to a subspace by the equation X^2^ + Y^2^ + Z^2^ = r^2^, were r is the radius of the sphere. Give a model which can describe points and lines on the surface of a sphere with less than 3 dimensions; i.e., define a space for the surface of a sphere with fewer than 3 dimensions.
The problems with trying to do this by defining a conformal map from 2 dimensional projective spaces to 3 dimensional surfaces is the reason whole books are written about projective geometry.
This doesn't make sense. Which projection? The natural one? Such a map is guaranteed to not be a bijection and is potentially not well-defined. Without a clear way of doing this map, you can't say anything about what happens to lines under the image of such a map.
I agree with this at least, I too am tired of the mathematical platonism dominating the public discourse.
latitude and longitude
just put the poles wherever it's convenient
idk, to me this is kinda semantics, whether or not we say a 2d surface in 3d space is 2d or 3d.
just assume "straight line" refers to a geodesic on the surface, then you can use whatever coordinates you want.